It should be fairly clear by now that the mysteries of physics have to do with "regimes" outside of our everyday experience. In addition to the regime of the very small, there are mysteries which involve the very large (described by General Relativity and Cosmology) and the very fast (Special Relativity when speeds approach the speed of light) which are outside the scope of this text. Our primary purpose in studying nuclear physics is to understand radioactivity, but we will take as many opportunities as possible to mention the things which make physics interesting and fun: the things which we do not yet understand!
We have actually already discussed the implications of the Rutherford Model in the last chapter, when we started with a model of the atom as a tiny solar system. We need to pursue the model a little further here in order to place the nucleus of the atom correctly in our intuition. Note that we are ignoring the chronology of the development of these theories in favor of a presentation which moves to progressively smaller, more "fundamental" phenonema.
The prevailing theory of the structure of the atom before Rutherford's experiment was the Thomson ("plum pudding") model of the atom, in which positive and negative charges were thought to be evenly distributed in a sphere (like raisins in plum pudding). To test this model, Rutherford fired columnated "alpha" particles (Helium nucleii travelling in a column) from 226Ra (see Section C below) at gold foil. Most went straight through, but some scattered at acute angles: a result "as surprising as if you were to fire cannon balls at tissue paper and have them bounce back at you".
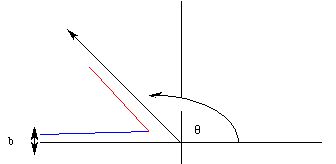
Imagine the nucleus to be at the origin and the alpha particles to be traveling in the x direction along the line y = b (b is called the "impact parameter"). The measured quantities in the experiment were the asymptotic angles at which the alpha particle bounced (was "scattered") from the nucleus as a function of the impact parameter:

| b (10 - 15m) | q (degrees) |
|---|---|
| 40.5 | 40 |
| 17.6 | 80 |
| 8.5 | 120 |
| 2.6 | 160 |
The analysis of the distribution of these "scattering angles" tells us a number of things about the atom.
The most obvious conclusion is that the structure of the atom mimics the structure of the solar system. In order to approximate the size of the nucleus at the center, we can equate the initial kinetic energy to the potential energy at the point of closest approach
of the alpha particles to find the distance of their closest approach (recall Section 2E). With 7.7 MeV (million electron volts) alpha particles, it is 2.95 x 10 - 14 m; therefore the nuclear radius is on the order of 10 - 15 m (1 "fermi", denoted F). Knowing that most of the mass of the atom is located in the nucleus, we find the nuclear density to be on the order of 10 18 kg / m 3. This implies that the positively charged protons in the nucleus must be very strongly attracted to each other in order to overcome the Coulomb repulsion. We also see that this attraction must be of short range, since the alpha particles were not captured, and that it must be independent of electric charge since neutrons are also held in the nucleus. This "strong" interaction is one of the four known fundamental interactions that we will meet in Section F.
The next section is about nuclear reactions.
If you have stumbled on this page, and the equations look funny (or you just want to know where you are!), see the College Physics for Students of Biology and Chemistry home page.
©1996, Kenneth R. Koehler. All Rights Reserved. This document may be freely reproduced provided that this copyright notice is included.
Please send comments or suggestions to the author.