Perhaps the single most important idea in physics is the idea of "conservation". A quantity which is "conserved" is unchanged by a process. This means that we do not have to know the details of the process to understand some of its consequences. There are a number of conserved quantities which we will deal with: mass, energy, momentum, electric charge. Each is conserved because the physics equations which we use to describe them has a "symmetry": there is some transformation which leaves the equation unchanged. So a quantity is conserved within measurable accuracy when there is an equation which accurately describes those measurements and which possess some symmetry.
For example, momentum is conserved in collisions. We express this conservation "law" as an equation:
Here P is a vector, the equation is actually a separate conservation equation for each direction independently, and the sum is over all of the objects involved in the collision. The above equation, when applied to two objects colliding in two dimensions, is equivalent to
and
In these equations, the number refers to the object and the letter refers to the component of the vector. Momentum is conserved in collisions because the forces acting during the collisions are purely internal to the colliding objects. The definition of force as the time rate of change of momentum, in the absence of external forces, becomes
This equation says that the momentum is a constant over time; that is, the equation is "symmetric with respect to the passage of time". Hence we do not need to know the details of the collision in order to understand the results of it in terms of the momenta of the objects involved. Consider the following collision:

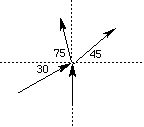
The numbers refer to angles relative to the coordinate axes (remember that we must establish a coordinate system to analyze any vector problem). Assume that the objects are both of mass 1 kg, and the object approaching from the left (#1) is moving at 2 m / s, while the one approaching from the bottom (#2) is moving at 1 m / s. The problem is to find their final speeds.
The momenta are
and
The conservation equations are then
and
These have the solution
and
In those situations where there is no friction, the total energy is also conserved. We usually write this as
This is a scalar equation. It comes from considering force as the spatial derivative of energy. If the external forces are zero, the total energy does not change from one location to the next. As an example, consider pushing your 90 kg professor off the top of the 30 m tall physics building. Initially, his kinetic energy is zero and his potential energy is m g h. Just before he hits the ground, all of his energy is kinetic, and his potential energy is zero. The conservation equation is then
This allows us to compute his final speed:
Note that his mass was not a factor in his final velocity. More importantly, note that we had to ignore friction in order to conserve energy.
Recall problem 2-1. We can understand the motion of a particle in an arbitrary potential by realizing that in the absence of friction, the total energy is a constant. Hence as a particle moves to a position of lower potential energy, its kinetic energy increases. When its potential energy is zero, its kinetic energy is enough to allow it to move into a region of higher potential energy. But as it does so, its kinetic energy decreases. This explains, for example, the oscillatory behavior of a particle in a quadratic potential. Similarly, when a particle has enough kinetic energy to approach a repulsive potential (where the potential energy increases as the particle moves towards the "source" of the potential), the kinetic energy decreases as the potential energy increases. The point of closest approach is the point where the difference between the initial kinetic and potential energies equals the current potential energy: all of the initial kinetic energy has "turned into" potential energy. Thus when a particle's initial position can be taken to have zero potential energy, we say that the distance of closest approach is when the initial kinetic energy is equal to the "final" potential energy.
Sometimes some of the energy in a system will be put to work. In situations when the work consists of a constant force applied through a distance Dx (ie., pushing an object), the work is
Note that work has dimensions of energy. Note also that if the object does not move, you have done no work (regardless of the amount of pushing you did!). On other occasions, work may be done against friction, and this allows us to incorporate friction into our conservation equation:
Here W is the work done by the system: against friction, or simply to accomplish something!
Conservation of mass and charge will be axioms for our purposes: we will simply state that mass and charge cannot be created or destroyed.
The next section is about stress and strain.
If you have stumbled on this page, and the equations look funny (or you just want to know where you are!), see the College Physics for Students of Biology and Chemistry home page.
©1996, Kenneth R. Koehler. All Rights Reserved. This document may be freely reproduced provided that this copyright notice is included.
Please send comments or suggestions to the author.